

Optimization and Systems Theory Seminar
Friday, December 8, 2000, 11.00-12.00, Room 3721, Lindstedtsv. 25
Jarmo Malinen
Division of Optimization and Systems Theory
Department of Mathematics
KTH
E-mail: jarmo.malinen@math.kth.se
On 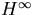 algebraic Riccati equations
algebraic Riccati equations
Let U, H and Y separable Hilbert spaces. Let 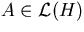 ,
, 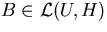 ,
,  ,
, 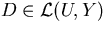 .We consider several spectral factorization, partial ordering and
invariant subspace problems, associated to an operator-valued
.We consider several spectral factorization, partial ordering and
invariant subspace problems, associated to an operator-valued
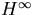 -transfer function
-transfer function
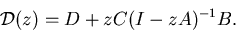
and certain solutions of an associated discrete time algebraic Riccati
equation

 Calendar of seminars
Calendar of seminars
Last update: November 30, 2000 by
Anders Forsgren,
anders.forsgren@math.kth.se.




 Calendar of seminars
Calendar of seminars